Context
This topic is part of the ANR project BECASIM (2013-2017). Using the home made software GPS (Gross Pitaevskii Simulator), we simulate rapidly rotating BEC with different kinds of trapping potentials.
BEC are modeled using the stationary Gross-Pitaevskii equation (in dimensionless form)$$\begin{array}{rcl} \mu\phi &=& -\frac12\Delta\phi + V(x)\phi + \beta |\phi|^2\phi - \Omega L_z\phi \\ \|\phi\|_{L^2} &=& 1. \end{array}$$ $\phi$ is the stationary wave function, $V$ is the magnetic trap, $\beta$ stands for the interaction between particles, $\Omega L_z$ is the angular momentum, and $\mu$ is the so-called chemical potential.
Tools
We use our home made software GPS:
- written in Fortran,
- hybrid MPI/OpenMP code,
- spatial discretization: spectral or high order compact finite differences scheme,
- imaginary time method with semi-implicit Crank-Nicolson scheme, or Sobolev gradient methods.
Results
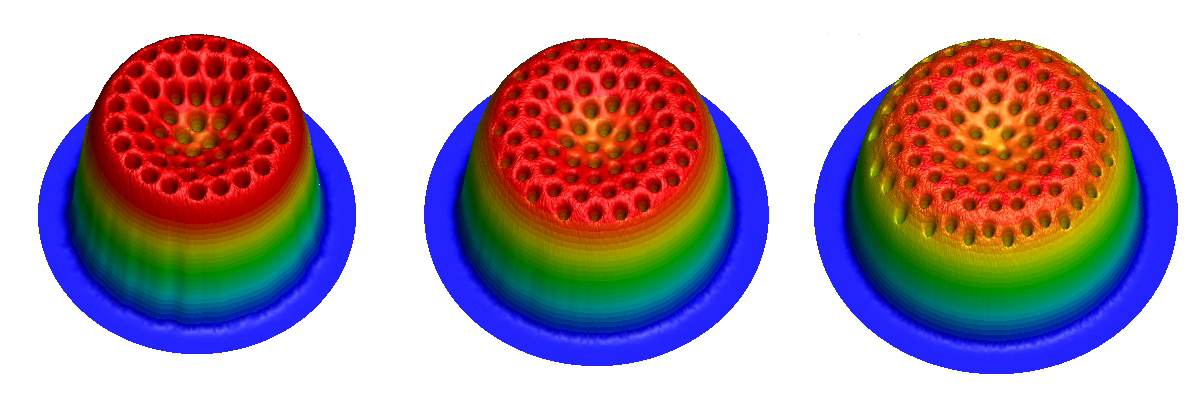Rotating BEC with different trapping potentials. Vortices are organized in Abrikosov lattices.